Negative mass
In theoretical physics, negative mass is a hypothetical concept of matter whose mass is of opposite sign to the mass of normal matter. Such matter would violate one or more energy conditions and show some strange properties such as being repelled rather than attracted by gravity. It is used in certain speculative theories, such as on the construction of wormholes. The closest known real representative of such exotic matter is a region of pseudo-negative pressure density produced by the Casimir effect.
Contents |
Inertial versus gravitational
Ever since Newton first formulated his theory of gravity, there have been at least three conceptually distinct quantities called mass: inertial mass, "active" gravitational mass (that is, the source of the gravitational field), and "passive" gravitational mass (that is, the mass that is evident from the force produced in a gravitational field). The Einstein equivalence principle postulates that inertial mass must equal passive gravitational mass; while the law of conservation of momentum requires that active and passive gravitational mass must be identical. All experimental evidence to date has found these are indeed always the same. In considering hypothetical particles with negative mass, it is important to consider which of these concepts of mass are negative; however, in most analyses of negative mass, it is assumed that the equivalence principle and conservation of momentum continue to apply.
In 1957, Hermann Bondi suggested in a paper in Reviews of Modern Physics that mass might be negative as well as positive.[1] He pointed out that this does not entail a logical contradiction, as long as all three forms of mass are negative, but that the assumption of negative mass involves some counter-intuitive form of motion.
From Newton's second law:
Thus it can be seen that an object with negative inertial mass would be expected to accelerate in the opposite direction to that in which it was pushed, which is arguably a strange concept. If the "push" is based on the electromagnetic force, this would simply mean the mass accelerates in the opposite direction than what one would expect based on its charge; for example, an object with negative inertial mass and positive charge would accelerate away from objects with positive mass and negative charge, and accelerate towards objects with positive mass and positive charge, the opposite of the normal rule that like charges repel and opposite charges attract.
If one were to treat inertial mass 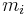 , passive gravitational mass
, passive gravitational mass  , and active gravitational mass
, and active gravitational mass 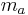 distinctly, then Newton's law of universal gravitation would take the form
distinctly, then Newton's law of universal gravitation would take the form
(where a is the acceleration of an object with inertial mass 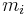 and passive gravitational mass
and passive gravitational mass  in the gravitational field generated by a different object with active gravitational mass
in the gravitational field generated by a different object with active gravitational mass 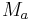 , with r as the distance between the two objects and G as the gravitational constant)
, with r as the distance between the two objects and G as the gravitational constant)
Thus objects with negative passive gravitational mass, but with positive inertial mass, would be expected to be repelled by positive active masses, and attracted to negative active masses. However, any difference between inertial and gravitational mass would violate the equivalence principle of general relativity. For an object where both the inertial and gravitational masses were negative and equal, we could cancel out 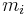 and
and  from the equation, and conclude that its acceleration a in the gravitational field from a body with positive active gravitational mass (say, the planet Earth) would be no different from the acceleration of an object with positive passive gravitational and inertial mass (so a small negative mass object would fall towards the Earth at the same rate as any other object).
from the equation, and conclude that its acceleration a in the gravitational field from a body with positive active gravitational mass (say, the planet Earth) would be no different from the acceleration of an object with positive passive gravitational and inertial mass (so a small negative mass object would fall towards the Earth at the same rate as any other object).
On the other hand, if we have a small object with equal inertial and passive gravitational masses falling in the gravitational field of an object with negative active gravitational mass (a small mass dropped above a negative-mass planet, say), then canceling out 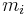 and
and  would indicate that the acceleration a of the small object is proportional to the negative active gravitational mass
would indicate that the acceleration a of the small object is proportional to the negative active gravitational mass 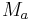 of the object creating the gravitational field, so the small object would actually accelerate away from the negative-mass object rather than towards it (and this is true regardless of whether the small object's inertial and passive gravitational masses were both positive or both negative). So, as long as inertial mass and gravitational mass are always equal as required by the equivalence principle, positive active gravitational mass would be universally attractive (both negative-mass and positive-mass objects would be pulled towards an object with positive active gravitational mass), while negative active gravitational mass would be universally repulsive (both negative-mass and positive-mass objects would be pushed away).
of the object creating the gravitational field, so the small object would actually accelerate away from the negative-mass object rather than towards it (and this is true regardless of whether the small object's inertial and passive gravitational masses were both positive or both negative). So, as long as inertial mass and gravitational mass are always equal as required by the equivalence principle, positive active gravitational mass would be universally attractive (both negative-mass and positive-mass objects would be pulled towards an object with positive active gravitational mass), while negative active gravitational mass would be universally repulsive (both negative-mass and positive-mass objects would be pushed away).
Forward's analysis
Although no particles are known to have negative mass, physicists (primarily Hermann Bondi and Robert L. Forward) have been able to describe some of the anticipated properties such particles may have. Assuming that all three concepts of mass are equivalent it would produce a system where negative masses are attracted to positive masses, yet positive masses are repelled away from negative masses. Negative masses would produce an attractive force on one another, but would move apart because of their negative inertial masses.
For a negative value of  with positive value of
with positive value of 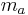 ,
,  is negative (repulsive); thus it would appear that a negative mass would accelerate away from a positive mass. But because such an object would also possess negative inertial mass it would accelerate in the opposite direction to
is negative (repulsive); thus it would appear that a negative mass would accelerate away from a positive mass. But because such an object would also possess negative inertial mass it would accelerate in the opposite direction to  . As Bondi pointed out, it can be shown that if both masses are of equal but opposite mass, the combined system of positive and negative particles will accelerate indefinitely without any additional input into the system.
. As Bondi pointed out, it can be shown that if both masses are of equal but opposite mass, the combined system of positive and negative particles will accelerate indefinitely without any additional input into the system.
This behavior is completely inconsistent with a common-sense approach and the expected behaviour of 'normal' matter; but is completely mathematically consistent and introduces no violation of conservation of momentum or energy. If the masses are equal in magnitude but opposite in sign, then the momentum of the system remains zero if they both travel together and accelerate together, no matter what their speed:
And equivalently for the kinetic energy 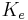 :
:
Forward extended Bondi's analysis to additional cases, and showed that even if the two masses m(-) and m(+) are not the same, the conservation laws remain unbroken.
This behaviour can produce bizarre results: for instance, a gas containing a mixture of positive and negative matter particles will have the positive matter portion increase in temperature without bound. However, the negative matter portion gains negative temperature at the same rate, again balancing out. Geoffrey A. Landis pointed out other implications of Forward's analysis,[2] including noting that although negative mass particles would repel each other gravitationally, the electrostatic force would be attractive for like-charges and repulsive for opposite charges.
Forward used the properties of negative-mass matter to create the diametric drive, a design for spacecraft propulsion using negative mass that requires no energy input and no reaction mass to achieve arbitrarily high acceleration.
Forward also coined a term, "nullification" to describe what happens when ordinary matter and negative matter meet: they are expected to be able to "cancel-out" or "nullify" each other's existence. An interaction between equal quantities of positive and negative mass matter would release no energy, but since the only configuration of such particles which has zero momentum (both particles moving with the same velocity in the same direction) does not produce a collision, all such interactions would leave a surplus of momentum, which is classically forbidden.
In general relativity
In general relativity, negative mass is generalized to refer to any region of space in which for some observers the mass density is measured to be negative. This can occur due to negative mass, or could be a region of space in which the stress component of the Einstein stress-energy tensor is larger in magnitude than the mass density. All of these are violations of one or another variant of the positive energy condition of Einstein's general theory of relativity; however, the positive energy condition is not a required condition for the mathematical consistency of the theory. (Various versions of the positive energy condition, weak energy condition, dominant energy condition, etc., are discussed in mathematical detail by Visser.[3])
Morris, Thorne and Yurtsever[4] pointed out that the quantum mechanics of the Casimir effect can be used to produce a locally mass-negative region of space-time. In this article, and subsequent work by others, they showed that negative matter could be used to stabilize a wormhole. Cramer et al. argue that such wormholes might have been created in the early universe, stabilized by negative-mass loops of cosmic string.[5] Stephen Hawking has proved that negative energy is a necessary condition for the creation of a closed timelike curve by manipulation of gravitational fields within a finite region of space;[6] this proves, for example, that a finite Tipler cylinder cannot be used as a time machine.
Gravitational interaction of antimatter
Virtually every modern physicist suspects that antimatter has positive mass and should be affected by gravity just like normal matter, although it is thought that this view has not yet been conclusively empirically observed.[7][8] It is difficult to directly observe gravitational forces at the particle level: at such small scales, electric forces tend to overwhelm gravitational interactions, especially since the methods of antimatter production currently in use typically generate very energetic particles. Furthermore, antiparticles must be kept separate from their normal counterparts or they will quickly annihilate. It is hoped that the ATRAP antimatter experiments will be able to make direct measurements.
Bubble chamber experiments are often cited as evidence that antiparticles have the same inertial mass as their normal counterparts, but a reversed electric charge. In these experiments, the chamber is subjected to a constant magnetic field which causes charged particles to travel in helical paths; the radius and direction of which correspond to the ratio of electric charge to inertial mass. Particle–antiparticle pairs are seen to travel in helices with opposite directions but identical radii, implying that the ratios differ only in sign; but this does not indicate whether it is the charge or the inertial mass which is inverted. However, particle–antiparticle pairs are observed to electrically attract one another, often as the prelude to annihilation. This behavior implies that both have positive inertial mass and opposite charges; if the reverse were true, then the particle with positive inertial mass would be repelled from its antiparticle partner.
References
- ^ H. Bondi (1957), "Negative Mass in General Relativity", Rev. Mod. Phys. 29 No. 3 July 1957, pp. 423ff
- ^ G. Landis, "Comments on Negative Mass Propulsion," J. Propulsion and Power, Vol. 7 No. 2, 304 (1991).
- ^ M. Visser (1995) Lorentzian Wormholes: from Einstein to Hawking, AIP Press, Woodbury NY, ISBN 1-56396-394-9
- ^ M. Morris, K. Thorne, and U. Yurtsever, Wormholes, Time Machines, and the Weak Energy Condition, Physical Review, 61, 13, September 1988, pp. 1446 - 1449
- ^ John G. Cramer, Robert L. Forward, Michael S. Morris, Matt Visser, Gregory Benford, and Geoffrey A. Landis, "Natural Wormholes as Gravitational Lenses," Phys. Rev. D51 (1995) 3117-3120
- ^ Hawking, Stephen (2002). The Future of Spacetime. W. W. Norton. pp. 96. ISBN 0-393-02022-3.
- ^ http://math.ucr.edu/home/baez/physics/ParticleAndNuclear/antimatter_fall.html
- ^ Antimatter FAQ
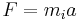
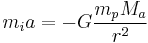
![P_{sys} = mv %2B (-m)v = [m%2B(-m)]v = 0\times v = 0.](/2012-wikipedia_en_all_nopic_01_2012/I/9c3272305b90639d8c8ce540202c8cd8.png)
![K_{e\ sys} = {1 \over 2} mv^2 %2B {1 \over 2}(-m)v^2 = {1 \over 2}[m%2B(-m)]v^2 = {1 \over 2}(0)v^2 = 0](/2012-wikipedia_en_all_nopic_01_2012/I/1985893e4d4009a8075934f615eae5f2.png)